Question

Darius and Barb are playing a video game in which the higher score wins the game. Their scores are shown below. Darius’s scores: 96, 54, 120, 87, 123 Barb’s scores: 92, 98, 96, 94, 110 Barb says that she is the winner. Darius says that it is a . Who is correct? Barb is correct if both the mean and median scores are considered. Barb is correct if only the median score is considered. Darius is correct if both the mean and median scores are considered. Darius is correct if only the median score is considered.

Answer 1

Answer: I think its D.

Explanation:

Im currently taking the quiz right now. Ill change it if its wrong.

Answer 2

D

Explanation:

Barb says shes the winner. Darius says its a tie. These claims are based off of the mean, the median, or both. Calculate the mean and find the median to find the right answer.

Darius

The mean is 96, and the median is 96.

`(96 +54+120+87+123)/(5) =96`

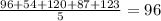
54 87 96 120 123

Barb

The mean is 98, and the median is 96.

`(92+98+96+94+110)/(5)=98`

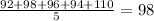
92 94 96 98 110

Now, look at the answer choices and pick the one that matches the results.

A) Wrong. Barb is correct if only the means is considered. She has a larger mean but an equal median.

B) Wrong. If the median is only considered, then Darius is correct, not Barb.

C) Wrong. Darius is only correct if the median is considered. If both are considered, then there is conflict.

D) Correct. Darius is correct of only the median is considered.