Question

If a monopolist supplies goods at a price; P = 170 - Q, with marginal cost; MC = 52. Find the quantity and price

Answer 1

Quantity = 59 units

Price = $111

Explanation:

The Demand function is given by

`P = 170 - Q`

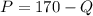
The Marginal cost is given by

`MC = 52`

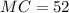
We are asked to find the quantity and price of goods.

Firstly, obtain the Marginal revenue function from the demand function

The Total revenue is given by

`TR = P * Q \\\\TR = (170 - Q) * Q \\\\TR = 170Q - Q^2`

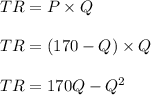
The Marginal revenue is the derivative of the Total revenue,

`MR = (d)/(dQ) (TR) = 170Q - Q^2 \\\\MR = (d)/(dQ) (TR) = 170 - 2Q \\\\`

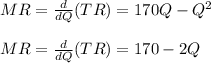
Assuming that the monopolist maximizes profits,

`MR = MC \\\\170 - 2Q = 52\\\\2Q = 170 - 52\\\\2Q = 118\\\\Q = 118/2\\\\Q = 59`

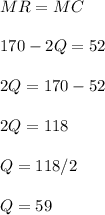
Therefore, the quantity is 59 units.

The price of each good is

`P = 170 - Q \\\\P = 170 - 59 \\\\P = 111`

Therefore, the price is $111.