Question

In 2000, a study of HCC algebra students says that 65% of them pass on their first attempt. In 2004, a survey of 450 algebra students reported that 287 passed on their first attempt. Is there sufficient evidence to suggest that the number of students that passed in 2004 is different than in 2000

Answer 1

The calculated value Z = 0.549 < 1.96 at 0.05 level of significance

Null hypothesis is accepted

There is no difference between the number of students that passed in 2004 and 2000

Explanation:

Step(i):-

Given Population proportion 'P' = 65% or 0.65

Given survey of 450 algebra students reported that 287 passed on their first attempt.

Sample proportion

`p = (x)/(n) = (287)/(450) = 0.6377`

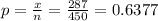
Null Hypothesis :H₀: The number of students that passed in 2004 is equal to 2000

Alternative Hypothesis :H₁: The number of students that passed in 2004 is different than in 2000

Step(ii):-

Test statistic

`Z = \frac{p - P}{\sqrt{(PQ)/(n) } }`

`Z = \frac{0.6377 - 0.65}{\sqrt{(0.65 x 0.35)/(450) } }`

`Z = (-0.0123)/(0.0224) = -0.549`

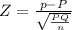
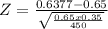
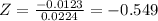
|Z| = |-0.549| = 0.549

The critical value

z₀.₀₅ = 1.96

The calculated value Z = 0.549 < 1.96 at 0.05 level of significance

Final answer:-

Null hypothesis is accepted

There is no difference between the number of students that passed in 2004 and 2000