Question

Ten years ago 53% of American families owned stocks or stock funds. Sample data collected by the Investment Company Institute indicate that the percentage is now 44%. (a) Choose the appropriate hypotheses such that rejection of H0 will support the conclusion that a smaller proportion of American families own stocks or stock funds this year than 10 years ago. H0: p - Select your answer - Ha: p - Select your answer - (b) Assume the Investment Company Institute sampled 300 American familie

Answer 1

a) The null and alternative hypothesis are:

`H_0: \pi=0.53\\\\H_a:\pi<0.53`

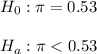
b) If 300 families were sampled, for a significance level of 5%, there is enough evidence to support the claim that a smaller proportion of American families own stocks or stock funds this year than 10 years ago (P-value = 0.001).

Explanation:

The claim that we want to have evidence to support is that a smaller proportion of American families own stocks or stock funds this year than 10 years ago.

The hypothesis for this test should state:

- For the null hypothesis, that the population proportion is not significantly different from 53%.

`H_0:\pi=0.53`

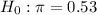
- For the alternative hypothesis, that the population proportion is significantly less than 53%.

`H_a: \pi<0.53`

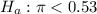
If 300 families are sampled, we can perform a hypothesis test for a proportion.

The claim is that a smaller proportion of American families own stocks or stock funds this year than 10 years ago.

Then, the null and alternative hypothesis are:

`H_0: \pi=0.53\\\\H_a:\pi<0.53`

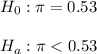
The significance level is 0.05.

The sample has a size n=300.

The sample proportion is p=0.44.

The standard error of the proportion is:

`\sigma_p=\sqrt{(\pi(1-\pi))/(n)}=\sqrt{(0.53*0.47)/(300)}\\\\\\ \sigma_p=√(0.00083)=0.029`

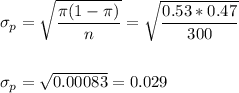
Then, we can calculate the z-statistic as:

`z=(p-\pi+0.5/n)/(\sigma_p)=(0.44-0.53+0.5/300)/(0.029)=(-0.088)/(0.029)=-3.065`

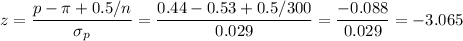
This test is a left-tailed test, so the P-value for this test is calculated as:

`\text{P-value}=P(z<-3.065)=0.001`

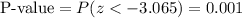
As the P-value (0.001) is smaller than the significance level (0.05), the effect is significant.

The null hypothesis is rejected.

There is enough evidence to support the claim that a smaller proportion of American families own stocks or stock funds this year than 10 years ago.