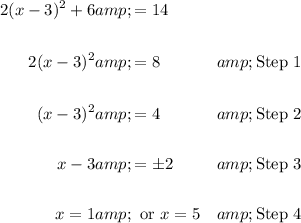Question

Tara solved a quadratic equation. Her work is shown below, with Step 222 missing. What could Tara have written as the result from Step 222? \begin{aligned} 2(x-3)^2+6&=14 \\\\ 2(x-3)^2&=8&\text{Step }1 \\\\ &&\text{Step }2 \\\\ x-3&=\pm 2&\text{Step }3 \\\\ x=1&\text{ or }x=5&\text{Step }4 \end{aligned} 2(x−3) 2 +6 2(x−3) 2 x−3 x=1 ​ =14 =8 =±2 or x=5 ​ Step 1 Step 2 Step 3 Step 4 ​

Answer 1

Step 2

Explanation:

I did the Khan Academy.

Answer 2

`(x-3)^2=4`

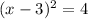
Explanation:

Tara's work is shown below:

`\begin{aligned} 2(x-3)^2+6&=14 \\\\ 2(x-3)^2&=8&\text{Step }1 \\\\ &&\text{Step }2 \\\\ x-3&=\pm 2&\text{Step }3 \\\\ x=1&\text{ or }x=5&\text{Step }4 \end{aligned}`

From the equation, we notice that in Step 1, Tara did:

`2(x-3)^2+6=14\\2(x-3)^2=14-6\\2(x-3)^2=8`

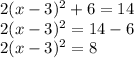
She is trying to isolate the x-variable. Therefore, the next logical step will be to divide both sides by 2 and her Step 2 will therefore be:

`(2(x-3)^2)/(2) =(8)/(2) \\\\(x-3)^2=4`

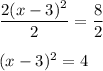
Tara could have written:
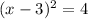`(x-3)^2=4` as her step 2 and we would then have her work as:

`\begin{aligned} 2(x-3)^2+6&=14 \\\\ 2(x-3)^2&=8&\text{Step }1 \\\\ (x-3)^2&=4&\text{Step }2 \\\\ x-3&=\pm 2&\text{Step }3 \\\\ x=1&\text{ or }x=5&\text{Step }4 \end{aligned}`