Question

The daily profit in dollars made by an automobile

manufacturer is

P(x) = -35x2 +2,100x - 20,000

where x is the number of cars produced per shift. Find the

maximum possible daily profit.

Answer 1

The maximum possible daily profit is $11,500.

Explanation:

Vertex of a quadratic function:

Suppose we have a quadratic function in the following format:

`f(x) = ax^(2) + bx + c`

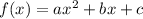
It's vertex is the point
`(x_(v), f(x_(v))`

In which

`x_(v) = -(b)/(2a)`

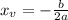
If a<0, the vertex is a maximum point, that is, the maximum value happens at
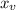`x_(v)`, and it's value is
`f(x_(v))`

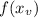
In this question:

The maximum daily profit happens when
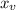`x_(v)` cars are sold. This profit is
`P(x_(v))`

`P(x) = -35x^(2) + 2100x - 20000`

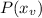
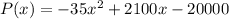
So
`a = -35, b = 2100`

`x_(v) = -(2100)/(2*(-35)) = 30`

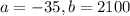
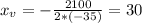
The maximum possible daily profit is:

`P(30) = -35*30^2 + 2100*30 - 20000 = 11500`

The maximum possible daily profit is $11,500.