Answer:
- The actual product is less than the estimate
- The numbers differ by less than one
Explanation:
Given
Product of 3 and two-fifths &

Required
Compare actual result with approximated result
Calculating the actual result;
3 and two-fifths means

So, the Product of 3 and two-fifths &
 becomes
becomes
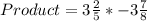
Convert fractions to improper
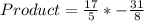
Combine fractions to form one
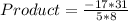
Multiply numerators and denominator

Convert fraction to decimal
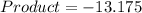
The nearest half of -13.175 is -13
Hence;
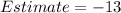
By comparison:-
- The actual product (-13.175) is less than the estimate (-13)
- The difference between both results is less than 1;
The difference is calculated as follows
Difference = |Actual Product - Estimate|
Difference = |-13.175 - (-13)|
Difference = |-13.175 +13|
Difference = |-0.175|
Difference = 0.175