Answer:
Quadrilateral
Explanation:
In the sandbox, opposite sides are equal.
However, using Pythagoras Theorem
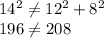
Therefore, the sandbox cannot be a rectangle since if it were, each triangle must be a right triangle.
In the triangle
- If
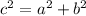 , C is a right angle
, C is a right angle - If
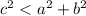 , C is an acute angle
, C is an acute angle - If
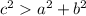 , C is an obtuse angle
, C is an obtuse angle
Since
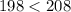 , Angles C and X are acute angles.
, Angles C and X are acute angles.
Therefore, the sandbox does not satisfy the properties of any known quadrilateral. It is simply a quadrilateral-shaped sandbox.