Question

The number of bacteria in a refrigerated food product is given by N ( T ) = 22 T 2 − 123 T + 40 , 6 < T < 36 , where T is the temperature of the food. When the food is removed from the refrigerator, the temperature is given by T ( t ) = 8 t + 1.7 , where t is the time in hours. Find the composite function N ( T ( t ) ) : N ( T ( t ) ) = Find the time when the bacteria count reaches 8019. Time Needed = hours

Answer 1

`N(T(t)) = 1408t^2 - 385.6t - 105.52`

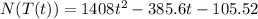
Time for bacteria count reaching 8019: t = 2.543 hours

Explanation:

To find the composite function N(T(t)), we just need to use the value of T(t) for each T in the function N(T). So we have that:

`N(T(t)) = 22 * (8t + 1.7)^2 - 123 * (8t + 1.7) + 40`

`N(T(t)) = 22 * (64t^2 + 27.2t + 2.89) - 984t - 209.1 + 40`

`N(T(t)) = 1408t^2 + 598.4t + 63.58 - 984t - 169.1`

`N(T(t)) = 1408t^2 - 385.6t - 105.52`

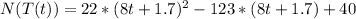
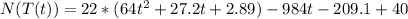
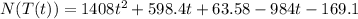
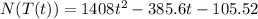
Now, to find the time when the bacteria count reaches 8019, we just need to use N(T(t)) = 8019 and then find the value of t:

`8019 = 1408t^2 - 385.6t - 105.52`

`1408t^2 - 385.6t - 8124.52 = 0`

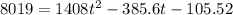
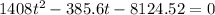
Solving this quadratic equation, we have that t = 2.543 hours, so that is the time needed to the bacteria count reaching 8019.