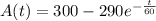Answer:
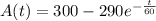
Explanation:
The volume of fluid in the tank = 300 Liters
Initial amount of Salt in the tank, A(0)=10 grams
Change in the Amount of Salt in the Tank
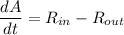
Rate In =(concentration of salt in inflow)(input rate of brine)
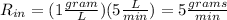
Rate Out =(concentration of salt in outflow)(output rate of brine)
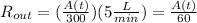
Therefore:
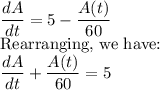
We solve the resulting linear differential equation for A(t)

Taking the integral of both sides
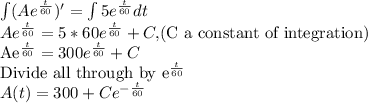
Recall that when t=0, A(t)=10 grams (our initial condition)
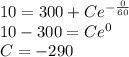
Therefore, the number A(t) of grams of salt in the tank at time t is: