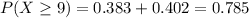Answer:
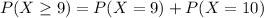
And using the probability mass function we got:
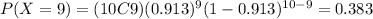
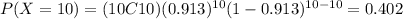
And replacing we got:
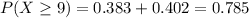
Explanation:
Let X the random variable of interest "number of students graduated", on this case we now that:
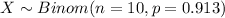
The probability mass function for the Binomial distribution is given as:
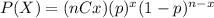
Where (nCx) means combinatory and it's given by this formula:

We want to find the following probability:
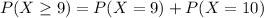
And using the probability mass function we got:
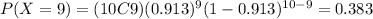
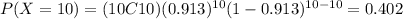
And replacing we got: