Answer:
a. Ff = 74.03N
b. a = 2.23 m/s^2
c. v = 9.73 m/s
Step-by-step explanation:
a. For an incline system, the force of kinetic friction is given by tte following formula:
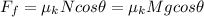 (1)
(1)
Ff: friction force
μk: kinetic friction = 0.123
N: normal force
θ: angle of the incline = 20.1°
M: mass of the student = 65.4 kg
g: gravitational acceleration = 9.8 m/s^2
You replace the values of the parameters in the equation (1):

The kinetic friction force is 74.03N
b. The acceleration of the student is given by the Newton second law:
 (2)
(2)
You solve the previous equation for a:
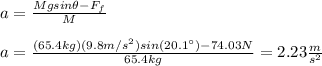
The acceleration of the student, in his trajectory is 2.23m/s^2
c. The speed of the student, at the bottom of the trajectory, can be calculated by taking into account that the net work is equal to the change in the kinetic energy:
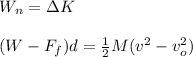 (3)
(3)
v: final speed = ?
vo: initial speed = 0 m/s
d: distance traveled by the student = 21.2m
Then, you replace the values of the parameters in the equation (3) and solve for v:
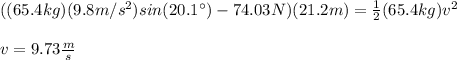
THe speed of the student at the bottom of the slide is 9.73 m/s