Answer:
37.27% probability that he or she will have a heart attack.
Explanation:
Bayes Theorem:
Two events, A and B.
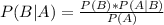
In which P(B|A) is the probability of B happening when A has happened and P(A|B) is the probability of A happening when B has happened.
In this question:
Event A: Periodontal disease
Event B: Heart attack
Researchers discovered that 82% of people who have suffered a heart attack had periodontal disease, an inflammation of the gums.
This means that
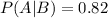
Only 33% of healthy people have this disease.
This means that
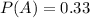
Suppose that in a certain community heart attacks are quite rare, occurring with only 15% probability.
This means that
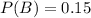
If someone has periodontal disease, what is the probability that he or she will have a heart attack
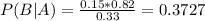
37.27% probability that he or she will have a heart attack.