Question

Suppose the speeds of vehicles traveling on a highway are normally distributed and have a known population standard deviation of 7 miles per hour and an unknown population mean. A random sample of 32 vehicles is taken and gives a sample mean of 64 miles per hour. Find the margin of error for the confidence interval for the population mean with a 98% confidence level.

Answer 1

2.879 (rounded 3 decimal places)

Explanation:

Answer 2

2.88

Explanation:

Data provided in the question

`\sigma` = Population standard deviation = 7 miles per hour

Random sample = n = 32 vehicles

Sample mean =
`\bar X` = 64 miles per hour

98% confidence level

Now based on the above information, the alpha is

= 1 - confidence level

= 1 - 0.98

= 0.02

For
`\alpha_1_2` = 0.01

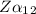`Z \alpha_1_2` = 2.326

Now the margin of error is

`= Z \alpha_1_2 * (\sigma)/(√(n))`

`= 2.326 * (7)/(√(32))`

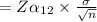
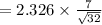
= 2.88

hence, the margin of error is 2.88