Answer:
The probability that the mean of this sample is less than 16.1 ounces of beverage is 0.0537.
Explanation:
We are given that the average amount of a beverage in randomly selected 16-ounce beverage can is 16.18 ounces with a standard deviation of 0.4 ounces.
A random sample of sixty-five 16-ounce beverage cans are selected
Let
 = sample mean amount of a beverage
= sample mean amount of a beverage
The z-score probability distribution for the sample mean is given by;
Z =
 ~ N(0,1)
~ N(0,1)
where,
 = population mean amount of a beverage = 16.18 ounces
= population mean amount of a beverage = 16.18 ounces
 = standard deviation = 0.4 ounces
= standard deviation = 0.4 ounces
n = sample of 16-ounce beverage cans = 65
Now, the probability that the mean of this sample is less than 16.1 ounces of beverage is given by = P(
 < 16.1 ounces)
< 16.1 ounces)
P(
 < 16.1 ounces) = P(
< 16.1 ounces) = P(
 <
<
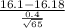 ) = P(Z < -1.61) = 1 - P(Z
) = P(Z < -1.61) = 1 - P(Z
 1.61)
1.61)
= 1 - 0.9463 = 0.0537
The above probability is calculated by looking at the value of x = 1.61 in the z table which has an area of 0.9591.