Answer:
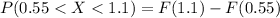
And replacing we got:
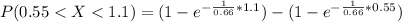
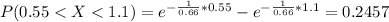
And rounded the answer would be 0.246
Explanation:
For this case we can define the random variable X as "The commute time for people in a city" and for this case the distribution of X is given by:
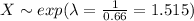
And for this case we want to find the following probability:
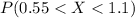
And we can use the cumulative distribution function given by:
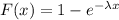
And using this formula we got:
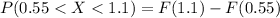
And replacing we got:
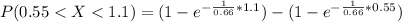
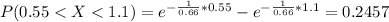
And rounded the answer would be 0.246