Answer:
The speed of the current is 3 mph.
Explanation:
The speed of he boat in calm water is "18 mph", the speed of the stream is "x" mph. When the boat is going downstream its speed is "x + 18", while when it goes upstream it will be "18-x". The time it takes for the boat to travel 21 miles downstream is:
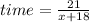
While the time it takes the boat to travel 15 miles upstream:
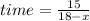
Since the times are the same, we can make:
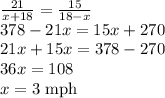
The speed of the current is 3 mph.