Question

We want to determine if the probability that a student enrolled in an accelerated math pathway is independent of whether the student is female. Which of the following pairs of probabilities is not a useful comparison?

a. 1360/2468 and 116/170
b. 170/2468 and 116/1360
c. 1360/2468 and 170/2468

Answer 1

The correct option is (c).

Explanation:

The complete question is:

The data for the student enrollment at a college in Southern California is:

Traditional Accelerated Total

Math-pathway Math-pathway

Female 1244 116 1360

Male 1054 54 1108

Total 2298 170 2468

We want to determine if the probability that a student enrolled in an accelerated math pathway is independent of whether the student is female. Which of the following pairs of probabilities is not a useful comparison?

a. 1360/2468 and 116/170

b. 170/2468 and 116/1360

c. 1360/2468 and 170/2468

Solution:

If two events A and B are independent then:

`P(A|B)=P(A)\\\\\&\\\\P(B|A)=P(B)`

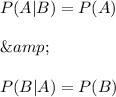
In this case we need to determine whether a student enrolled in an accelerated math pathway is independent of the student being a female.

Consider the following probabilities:

`P (F|A) = \farc{116}{170}\\\\P(A|F)=(116)/(1360)\\\\P(A)=(170)/(2468)\\\\P(F)=(1360)/(2468)`

If the two events are independent then:

P (F|A) = P(F)

&

P (A|F) = P (A)

But what would not be a valid comparison is:

P (A) = P(F)

Thus, the correct option is (c).