Question

A 397-N wheel comes off a moving truck and rolls without slipping along a highway. At the bottom of a hill it is rotating at 25.0 rad/s. The radius of the wheel is 0.600 m, and its moment of inertia about its rotation axis is 0.800MR2. Friction does work on the wheel as it rolls up the hill to a stop, a height h above the bottom of the hill; this work has absolute value 2500 J. Calculate h in meters.

Answer 1

h = 14.4 m

Step-by-step explanation:

The height can be calculated by energy conservation:

`K_(r) + K_(t) - W = E_(p)`

Where:

W: is the work

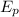`E_(p)`: is the potential energy

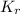`K_(r)`: is the rotational kinetic energy

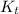`K_(t)`: is the transitional kinetic energy

Initially, the wheel has rotational kinetic energy and translational kinetic energy, and then when stops it has potential energy.

`K_(r) + K_(t) - W = E_(p)`

`(1)/(2)I\omega_(0)^(2) + (1)/(2)mv^(2) - W = mgh`

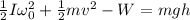
Where:

I: is the moment of inertia = 0.800 mr²

ω₀: is the angular speed = 25.0 rad/s

m: is the mass = P/g = 397 N/9.81 m*s⁻² = 40.5 kg

v: is the tangential speed = ω₀r²

Now, by solving the above equation for h we have:

`h = ((1)/(2)(I\omega_(0)^(2) + mv^(2)) - W)/(mg)`

`h = ((1)/(2)(I\omega_(0)^(2) + m(\omega_(0)*r)^(2)) - W)/(mg)`

`h = ((1)/(2)(0.800*40.5 kg*(0.600 m)^(2)*(25.0 rad/s)^(2) + 40.5 kg*(25.0 rad/s*0.600 m)^(2)) - 2500 J)/(40.5 kg*9.81 m/s^(2)) = 14.4 m`

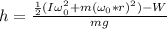
Therefore, the height is 14.4 m.

I hope it helps you!