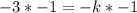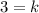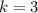Answer:
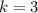
Explanation:
Given

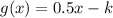
Required
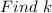
Initial expression of f(x) is

From the question; we understand that f(x) was shifted down by 3 units;
This implies that
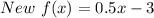
Also from the question; we understand that this new f(x) is equivalent to g(x);
In other words;
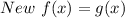
Substitute
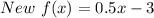 and
and
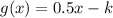
This gives
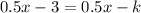
Subtract 0.5x to both sides

Rearrange
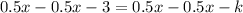
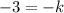
Multiply both sides by -1