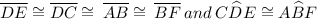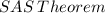Answer:
Check below please.
Explanation:
Hi,
Let's plot the figure, 1 square and 2 equilateral triangles.
1) Let's remember all the angles we already know, from the square and the equilateral triangle from their respective definition.
In other words:
Statement Reason
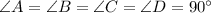 Given
Given

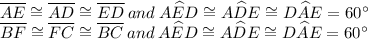
2) We have two triangles ABF and CDE
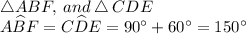
3) The Side, Angle Side Congruence Theorem assures us that both triangles are congruent. When there are two known legs (4 cm and 4 cm) of each triangle, and their respective formed angle is also known (150º). Therefore, these two triangles are both congruent.
Statement Reason