Question

In the previous part, we obtained dy dx = 3t2 − 27 −2t . Next, find the points where the tangent to the curve is horizontal. (Enter your answers as a comma-separated list of ordered pairs.)

x = t^3 - 3t, y = t^2 - 4

Answer 1

(27.55, 7.22), (-11.3, 3.21).

Explanation:

When is the tangent to the curve horizontal?

The tangent curve is horizontal when the derivative is zero.

The derivative is:

`(dy)/(dx) = 3t^(2) - 2t - 27`

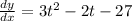
Solving a quadratic equation:

Given a second order polynomial expressed by the following equation:

`ax^(2) + bx + c, a\\eq0`.

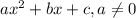This polynomial has roots
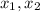`x_(1), x_(2)` such that
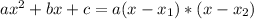`ax^(2) + bx + c = a(x - x_(1))*(x - x_(2))`, given by the following formulas:

`x_(1) = (-b + √(\bigtriangleup))/(2*a)`

`x_(2) = (-b - √(\bigtriangleup))/(2*a)`

`\bigtriangleup = b^(2) - 4ac`

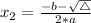
In this question:

`3t^(2) - 2t - 27 = 0`

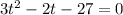
So

`a = 3, b = -2, c = -27`

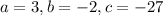
Then

`\bigtriangleup = b^(2) - 4ac = (-2)^(2) - 4*3*(-27) = 328`

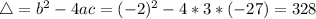
So

`t_(1) = (-(-2) + √(328))/(2*3) = 3.35`

`t_(2) = (-(-2) - √(328))/(2*3) = -2.685`

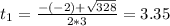
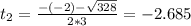
Enter your answers as a comma-separated list of ordered pairs.

We found values of t, now we have to replace in the equations for x and y.

t = 3.35

`x = t^(3) - 3t = (3.35)^(3) - 3*3.35 = 27.55`

`y = t^(2) - 4 = (3.35)^2 - 4 = 7.22`

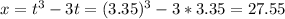
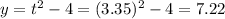
The first point is (27.55, 7.22)

t = -2.685

`x = t^(3) - 3t = (-2.685)^3 - 3*(-2.685) = -11.3`

`y = t^(2) - 4 = (-2.685)^2 - 4 = 3.21`

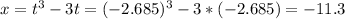
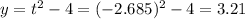
The second point is (-11.3, 3.21).