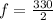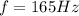Answer:
The frequency is
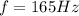
Step-by-step explanation:
From the question we are told that
The position of zero intensity is
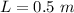 from the center
from the center
Now the wavelength of the sound is mathematical represented as

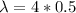
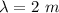
Now the frequency of the sound is mathematically represented as
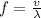
substituting values