Answer:
1.) .800
2.).779 to .821
Explanation:
We must assume that the sample that we are given to work with is representative of the population, in which case we just need to find the proportion of people in the sample who don't smoke. This is
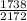 = .8002, or .800 rounded to three decimal places.
= .8002, or .800 rounded to three decimal places.
Part 2 will build off the answer in step 1. The middle of the confidence interval will be the estimate gained from the sample, or .800. The critical value of 98% equals 2.326 when using the normal model. The standard deviation can be calculated using the formula
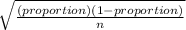 . Plugging in the numbers you get
. Plugging in the numbers you get
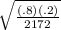 = .009.
= .009.
This means the confidence interval is .800±.021