Question

For the function, find all critical numbers and then use the second-derivative test to determine whether the function has a relative maximum or minimum at each critical number. (Enter your answers as a comma-separated list. If an answer does not exist, enter DNE.)

f(x) = x3 − 12x2 + 21x − 8relative maxima x=relative minima x=

Answer 1

relative maximum: x = 1

relative minimum: x = 7

Explanation:

Critical points:

Values of x for which f'(x) = 0.

Second derivative test:

For a critical point, if f''(x) > 0, the critical point is a relative minimum.

Otherwise, if f''(x) < 0, the critical point is a relative maximum.

Solving a quadratic equation:

Given a second order polynomial expressed by the following equation:

`ax^(2) + bx + c, a\\eq0`.

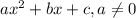This polynomial has roots
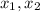`x_(1), x_(2)` such that
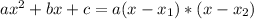`ax^(2) + bx + c = a(x - x_(1))*(x - x_(2))`, given by the following formulas:

`x_(1) = (-b + √(\bigtriangleup))/(2*a)`

`x_(2) = (-b - √(\bigtriangleup))/(2*a)`

`\bigtriangleup = b^(2) - 4ac`

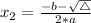
In this question:

`f(x) = x^(3) - 12x^(2) + 21x - 8`

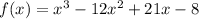
Finding the critical points:

`f'(x) = 3x^(2) - 24x + 21`

`3x^(2) - 24x + 21 = 0`

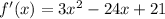
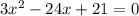
Simplifying by 3

`x^(2) - 8x + 7 = 0`

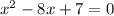
So
`a = 1, b = -8, c = 7`

`\bigtriangleup = (-8)^(2) - 4*1*7 = 36`

`x_(1) = (-(-8) + √(36))/(2) = 7`

`x_(2) = (-(-8) - √(36))/(2) = 1`

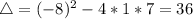
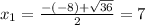
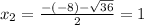
Second derivative test:

The critical points are x = 1 and x = 7.

The second derivative is:

`f''(x) = 6x - 24`

`f''(1) = 6*1 - 24 = -18`

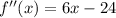
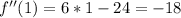
Since f''(1) < 0, at x = 1 there is a relative maximum.

`f''(7) = 6*7 - 24 = 18`

Since f''(x) > 0, at x = 7 there is a relative minumum.