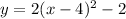Answer:


Explanation:
Vertex form of a parabola:

(where (h, k) is the vertex and
 is some constant)
is some constant)
Part a
- Vertex = (-1, -4)
- Point on parabola = (1, 0)
Substitute the vertex into the formula:
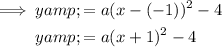
Substitute the point (1, 0) into the formula:
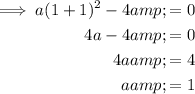
Therefore, the equation of the parabola in vertex form is:
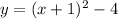
Part b
- Vertex = (4, -2)
- Point on parabola = (6, 6)
Substitute the vertex into the formula:
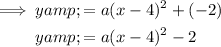
Substitute the point (6, 6) into the formula:
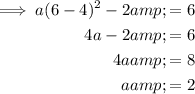
Therefore, the equation of the parabola in vertex form is: