Question

The first three excited states of the nucleus Au-199 (gold) are at 0.075 Mev, 0.320 Mev and 0.475 MeV. If all transitions between theses states and the ground state occurred, what energy/wavelength gamma rays would be observed?

Answer 1

Answer and Explanation:

The computation of the energy or wavelength gamma rays observed is shown below:

Since the energy of gamma rays is higher than 0.10 MeV.

Now We have to calculate transitions in between the given levels of energy that correspond to this energy.

As per the given question, we have the following information

Ground state = E where E < 0.075 MeV

For Level 1 = 0.075 MeV

For Level 2 = 0.320 MeV

For Level 3 = 0.475 MeV

Now we have to take the below transitions:

1.
`3 \rightarrow 2`

Difference of energy is

= 0.475 - 0.320

= 0.155 MeV

This represents a gamma radiation

2.
`3 \rightarrow 1`

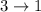
Difference of energy is

= 0.475 - 0.075

= 0.4 MeV

This represents a gamma radiation

3.
`3 \rightarrow ground`

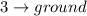
Difference of energy is

= 0.475 - E > 0.155 MeV

This represents a gamma radiation

4.
`2 \rightarrow 1`

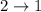
Difference of energy is

= 0.320 - 0.075

= 0.245 MeV

This represents a gamma radiation

5.
`2 \rightarrow ground`

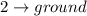
Difference of energy is

= 0.320 - E > 0.245 MeV

This represents a gamma radiation

6.
`1 \rightarrow Ground`

Difference of energy is

= 0.075 - E < 0.10 MeV

This represents not a gamma radiation

We can see that there are 5 transitions that contain gamma rays