Question

3

`3 \sqrt{54a {8y {5 / 3 \sqrt{2a { - 1y {2}^(?) }^(?) } }^(2) }^(2) }`
​

Answer 1

(a³y 3√20u)/2

Explanation :

I used these steps in solving the question

I hope it helps :)

1. Note : The quotient of roots with the same indexis equal to the root of the quotient
2. Write the division as a fraction
3. Simply the expression
4. 
   `\frac{ {a}^(8) }{ {a}^( - 1) } = {a}^(9) \\ \frac{ {y}^(5) }{ {y}^(2) } = {y}^(3)`
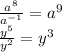
5. Use the communative property to reorder the terms
6. Take the root of the numerator and denominator separately.
7. Simplify the radical expression
8. Multiply the fraction by
9. 
   `\frac{ \sqrt[3]{ {2}^(2) } }{ \sqrt[3]{ {2}^(2) } }`
10. Calculate the product
11. Evaluate the power ( in numerator)
12. Calculate the product ( in denominator)
13. Calculate the product of 5×4u
14. Reduce the index of the radical and exponents with 3