Question

Triangles R S T and V U T are connected at point T. Angles R S T and V U T are right angles. The length of side R S is 12 and the length of side S T is 16. The length of side T U is 8 and the length of U V is 6. Which statements can be concluded from the diagram and used to prove that the triangles are similar by the SAS similarity theorem? StartFraction R S Over V U EndFraction = StartFraction S T Over U T EndFraction and angle S is-congruent-to angle U StartFraction R S Over V U EndFraction = StartFraction S T Over U T EndFraction = StartFraction R T Over V T EndFraction StartFraction R S Over V U EndFraction = StartFraction T U Over T S EndFraction and angle S is-congruent-to angle U StartFraction R S Over V U EndFraction = StartFraction T U Over T S EndFraction = StartFraction R T Over V T EndFraction

Answer 1

RS/VU=ST/UT and Angle S congruent Angle U

Answer 2

`\frac{\overline{RS}}{\overline{VU}}=\frac{\overline{ST}}{\overline{UT}}\quad\text{and}\quad\angle S\cong\angle U`

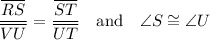
Explanation:

To use the SAS similarity theorem, you must show proportionality between corresponding sides, and congruence of the angle between them.

The answer shown above is the only answer choice that mentions an angle.

__

Comment on other choices

Choice B shows the three sides are proportional, so would be useful if the SSS similarity theorem were to be invoked. It isn't helpful for the using the SAS similarity theorem.

Choices C and D get the proportion statements wrong.