Answer:
The distance between midpoint of AP and QB is
 .
.
Explanation:
Given that: AB = a
AP = 2PQ = 2QB
Thus,
PQ = QB =
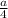
PQ + QB =
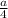 +
+
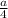
=
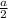
AP =
 a =
a =
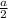
The distance between midpoint of AP and QB can be determined as;
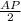 + PQ +
+ PQ +

=
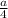 +
+
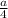 +
+
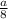
=
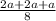
=

Therefore, the distance between midpoint of AP and QB is
 .
.