Answer:
SA = 169 feet
Explanation:
Surface Area of triangular prism = b₁h₁+2b₂h₂ + b₃h₃
Where b₁ is the base of triangular side(6 ft), h₁ is the height of triangular side (4 ft), b₂ is the base of rectangular side (9 ft), h₂ is the height of rectangular side(5 ft), b₃ is the base of the base(6 ft), h₃ is the height of the base(4 ft).
SA =
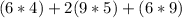
SA = 24+90+54
SA = 169 feet