Answer:
Choice A: approximately
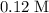 .
.
Step-by-step explanation:
Note that the unit of concentration,
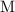 , typically refers to moles per liter (that is:
, typically refers to moles per liter (that is:
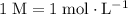 .)
.)
On the other hand, the volume of the two solutions in this question are apparently given in
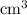 , which is the same as
, which is the same as
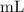 (that is:
(that is:
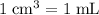 .) Convert the unit of volume to liters:
.) Convert the unit of volume to liters:
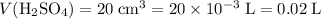 .
.
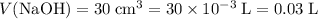 .
.
Calculate the number of moles of
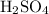 formula units in that
formula units in that
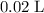 of the
of the
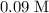 solution:
solution:
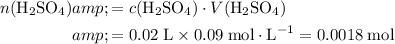 .
.
Note that
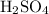 (sulfuric acid) is a diprotic acid. When one mole of
(sulfuric acid) is a diprotic acid. When one mole of
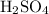 completely dissolves in water, two moles of
completely dissolves in water, two moles of
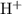 ions will be released.
ions will be released.
On the other hand,
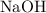 (sodium hydroxide) is a monoprotic base. When one mole of
(sodium hydroxide) is a monoprotic base. When one mole of
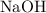 formula units completely dissolve in water, only one mole of
formula units completely dissolve in water, only one mole of
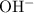 ions will be released.
ions will be released.
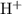 ions and
ions and
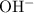 ions neutralize each other at a one-to-one ratio. Therefore, when one mole of the diprotic acid
ions neutralize each other at a one-to-one ratio. Therefore, when one mole of the diprotic acid
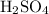 dissolves in water completely, it will take two moles of
dissolves in water completely, it will take two moles of
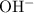 to neutralize that two moles of
to neutralize that two moles of
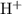 produced. On the other hand, two moles formula units of the monoprotic base
produced. On the other hand, two moles formula units of the monoprotic base
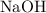 will be required to produce that two moles of
will be required to produce that two moles of
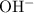 . Therefore,
. Therefore,
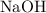 and
and
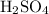 formula units would neutralize each other at a two-to-one ratio.
formula units would neutralize each other at a two-to-one ratio.
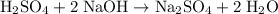 .
.
 .
.
Previous calculations show that
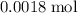 of
of
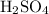 was produced. Calculate the number of moles of
was produced. Calculate the number of moles of
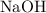 formula units required to neutralize that
formula units required to neutralize that
 .
.
Calculate the concentration of a
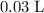 solution that contains exactly
solution that contains exactly
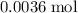 of
of
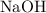 formula units:
formula units:
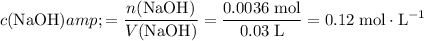 .
.