Question

Find the total surface area of the following prism

Answer 1

Surface area of the prism = 340 cm²

Explanation:

Surface area of the prism given in the figure,

Surface area = Two pentagonal bases of the prism + Area of the five lateral rectangular sides

Area of the pentagonal base =
`(1)/(2)* a * P`

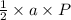
Here 'a' = apothem of the pentagon

P = Perimeter of the pentagon

P = 5×5 = 25 cm

a = 3.6 cm

Area of the pentagon =
`(1)/(2)(25)(3.6)`

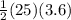
= 45 cm²

Area of a rectangular lateral side = Length × width

= 10 × 5

= 50 cm²

Area of the prism = 2(Area of pentagonal base) + 5(Area of one rectangular side)

= 2(45) + 5(50)

= 90 + 250

= 340 cm²

Therefore, surface area of the given prism = 340 cm²