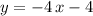Question

Choose the equation that satisfies the data in the table.

Answer 1

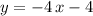`y=-4\,x-4` which agrees with answer "A" in the list of possible options

Explanation:

From the fact that there is a steady rate of increase in the x and in the respective y values (notice that x increases in steps of 1 unit, and at the same time y values increase in steps of "-4" units) the data belongs to the equation of a line.

We can determine that equation using just two pairs of points, like for example (-1, 0) and (0, -4).

We first find the slope using the slope formula for the segment that joints any two points on the plane:

`slope=(y_2-y_1)/(x_2-x_1) \\slope=(-4-0)/(0-(-1))\\slope = -4`

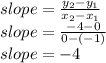
we now write the slope-intercept general form of a line with slope "-4", and solve for the intercept by using the point (0,-4) which should be in the line:

`y=m\.x+b\\y=-4\,x+b\\-4=-4\,(0)+b\\b=-4`

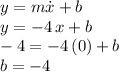
Therefore the equation for this points is:

`y=-4\,x-4`