Answer:
(x, y) - (y, -x)
Explanation:
Rotation is a process in which the orientation of a given figure is changed by turning it about a point called origin. The rotation can be done either clock-wisely or counterclockwise about the origin.
If the given triangle is rotated
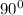 clockwise, the rule that describe the transformation is; (x, y) - (y, -x)
clockwise, the rule that describe the transformation is; (x, y) - (y, -x)
If the given triangle is rotated
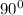 counterclockwise, the rule that describe the transformation is; (x, y) - (-y, x)
counterclockwise, the rule that describe the transformation is; (x, y) - (-y, x)
In the given question, the required rule is; (x, y) - (y, -x). This shows a clockwise rotation of
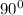 about the origin.
about the origin.