Answer: t = 9.05 min
Explanation: The rate of temperature over time is given by:
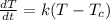
where:
K is the constant of transference of heat
 is temperature of the container
is temperature of the container
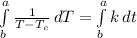
ln (T -
 ) = kt + c
) = kt + c
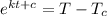
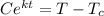

In container A, the temperature is at 0°C, so
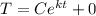
For the bar, when t = 0 min, T = 100°C:
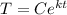
100 =

C = 100
After 1 minute, the temperature of the bar is 90°C, so:
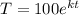
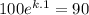
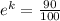

k = ln(0.9)
k = - 0.105

After 2 minutes, the temperature will be:

T = 81.06°C
For container B, the temperature is 100°C, so:

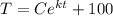
The initial temperature of the bar when entering the container B is T = 81.06°C, then:
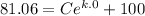
C = - 18.94
After 1 minute, the temperature rises 10°C:
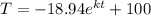
91 =
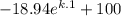
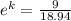
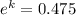
k = ln(0.475)
k = - 0.744
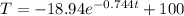
When T = 99.9°C:
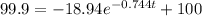
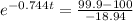
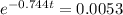
t =

t = 7.05 min
The entire process will take:
t = 2 + 7.05
t = 9.05 min