Answer:
The percentage is
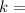 1.02%
1.02%
Step-by-step explanation:
From the question we are told that
The speed of the sprinter is
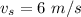
The radius of the curve is

The centripetal acceleration at the curve is mathematically represented as
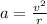
substituting values
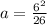
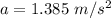
Now the force acting on the sprinter around the curve are
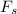 which represents centripetal force which is mathematically evaluated as
which represents centripetal force which is mathematically evaluated as

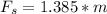
and
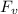 which is the centrifugal force which is generally represented as
which is the centrifugal force which is generally represented as
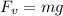
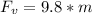
Now the resultant force which is the force acting on the sprinter when running around the curve is mathematically represented as

substituting values
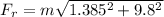
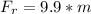
the average total force on their feet compared to when they are running in a straight line is mathematically evaluated as
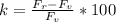
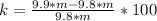
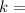 1.02%
1.02%