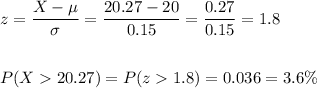Question

Ball-Bearings, Inc. produces ball bearings automatically on a Kronar BBX machine. For one of the ball bearings, the mean diameter is set at 20.00 mm (millimeters). The standard deviation of the production over a long period of time was computed to be 0.150 mm. What percent of the ball bearings will have diameters of 20.27 mm or more

Answer 1

3.6% of the ball bearings will have diameters of 20.27 mm or more.

Explanation:

We can calculate this proportion using the z-score.

We have a normal distribution with mean 20.00 mm and standard deviation of 0.150 mm.

The value for X is 20.27 mm.

Then, the z-score and its associated probability are:

`z=(X-\mu)/(\sigma)=(20.27-20)/(0.15)=(0.27)/(0.15)=1.8\\\\\\P(X>20.27)=P(z>1.8)=0.036=3.6\%`