Question

A 30-m-long rocket train car is traveling from Los Angeles to New York at 0.5c when a light at the center of the car flashes. When the light reaches the front of the car. it immediately rings a bell. Light reaching the back of the car immediately sounds a siren.

Part A
Are the bell and siren simultaneous events for a passenger seated in the car?
If not, which occurs first?
Yes, the bell and siren are simultaneous events. No, the siren sounds before the bell rings.
No, the bell rings before the siren sounds.
Part B
Are the bell and siren simultaneous events for a bicyclist waiting to cross the tracks?
If not, which occurs first?
Yes, the bell and siren are simultaneous events.
No, the siren sounds before the bell rings. No, the bell rings before the siren sounds.

Answer 1

Given that,

Distance =30 m

speed = 0.5c

(A). We need to find the bell and siren simultaneous events for a passenger seated in the car

According to given data

The distance travelled by the light to reach either side of the rocket train car is same.

So, The two events are simultaneous and the bell and siren are the simultaneous events for a passenger seated in the car.

(B). We need to calculate time interval between the events

Using formula of time dilation

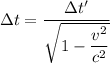`\Delta t=\frac{\Delta t'}{\sqrt{1-(v^2)/(c^2)}}`.....(I)

Where,
`\delta t'` = proper time

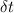`\delta t` = time interval between the events

The time interval between the events measured in a reference frame

The proper time in this case is

`\Delta t'=\Delta t_(1)-(v\Delta x)/(c^2)`

For the second interval,

Put the value of
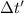`\Delta t'` in the equation (I)

`\Delta t_(2)=\frac{\Delta t_(1)-(v\Delta x)/(c^2)}{\sqrt{1-(v^2)/(c^2)}}`

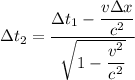
Put the value in the equation

`\Delta t_(2) = \frac{0-(0.5c*30)/(c^2)}{\sqrt{1-(0.5^2c^2)/(c^2)}}`

`\Delta t_(2)=(-15)/(3*10^(8)√(1-0.25))`

`\Delta t_(2)=-5.77*10^(8)\ s`

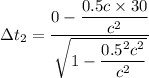
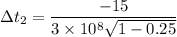
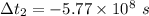
Negative sign shows the siren rings before the bell ring.

Hence, (A). Yes, the bell and siren are simultaneous events.

(B). The siren sounds before the bell rings.