Question

A car travels 13 km in a southeast direction and then 16 km 40 degrees north of east. What is the car's resultant direction?

Answer 1

21.48 km 2.92° north of east

Step-by-step explanation:

To find the resultant direction, we need to calculate a sum of vectors.

The first vector has module = 13 and angle = 315° (south = 270° and east = 360°, so southeast = (360+270)/2 = 315°)

The second vector has module 16 and angle = 40°

Now we need to decompose both vectors in their horizontal and vertical component:

horizontal component of first vector: 13 * cos(315) = 9.1924

vertical component of first vector: 13 * sin(315) = -9.1924

horizontal component of second vector: 16 * cos(40) = 12.2567

vertical component of second vector: 16 * sin(40) = 10.2846

Now we need to sum the horizontal components and the vertical components:

horizontal component of resultant vector: 9.1924 + 12.2567 = 21.4491

vertical component of resultant vector: -9.1924 + 10.2846 = 1.0922

Going back to the polar form, we have:

`module = √(horizontal^2 + vertical^2)`

`module = √(460.0639 + 1.1929)`

`module = 21.4769`

`angle = arc\ tangent(vertical/horizontal)`

`angle = arc\ tangent(1.0922/21.4491)`

`angle = 2.915\°`

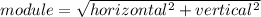
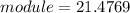
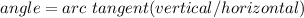
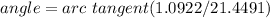
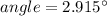
So the resultant direction is 21.48 km 2.92° north of east.