Question

The weekly mean income of a group of executives is $1,000 and the standard deviation of this group is $100. The distribution is normal. What percent of the executives have an income of $925 or less

Answer 1

23% percent of the executives have an income of $925 or less.

Explanation:

We have a normal distribution with mean 1,000 and standard deviation 100.

We have to calculate the proportion of exectutives that have an income of 95 or less. We can calculate this as the probability that X<925.

To do that, we calculate the z-score for X=925:

`z=(X-\mu)/(\sigma)=(925-1000)/(100)=(-75)/(100)=-0.75`

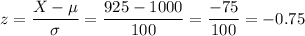
Then, with this value for the z-score we can calculate the probability of a randomy selected executive has a income of $925 or less (this value is equal to the proportion we want to calculate):

`P(X<925)=P(z<-0.75)=0.23`