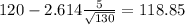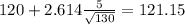Question

A random sample of 130 students is chosen from a population of 4,500 students. The mean IQ in the sample is 120, with a standard deviation of 5. Using a margin of error of 1.13%, what is the 99% confidence interval for the students' mean IQ score?

Answer 1

`120-2.614(5)/(√(130))=118.85`

`120+2.614(5)/(√(130))=121.15`

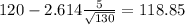
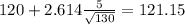
Explanation:

Information given

`\bar X= 120` represent the sample mean

`\mu` population mean (variable of interest)

s=5 represent the sample standard deviation

n=130 represent the sample size

For this case we can't set a margin of error just with a % since they not specify 1.13% respect to something for this case we can omit this value

Confidence interval

The confidence interval for the mean is given by the following formula:

`\bar X \pm t_(\alpha/2)(s)/(√(n))` (1)

The degrees of freedom are given by:

`df=n-1=130-1=129`

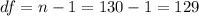
The Confidence level is 0.99 or 99%, the significance would be
`\alpha=0.01` and
`\alpha/2 =0.005`, and the critical value would be
`t_(\alpha/2)=2.614`

And replacing we got:

`120-2.614(5)/(√(130))=118.85`

`120+2.614(5)/(√(130))=121.15`