Question

Engineers want to design passenger seats in commercial aircraft so that they are wide enough to fit 95 percent of adult men. Assume that adult men have hip breadths that are normally distributed with a mean of 14.4 inches and a standard deviation of 1.1 inches. Find the 95th percentile of the hip breadth of adult men. Round your answer to one decimal place; add a trailing zero as needed. The 95th percentile of the hip breadth of adult men is [HipBreadth] inches.

Answer 1

`z=1.64<(a-14.4)/(1.1)`

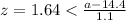
And if we solve for a we got

`a=14.4 +1.64*1.1=16.204`

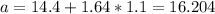
The 95th percentile of the hip breadth of adult men is 16.2 inches.

Explanation:

Let X the random variable that represent the hips breadths of a population, and for this case we know the distribution for X is given by:

`X \sim N(14.4,1.1)`

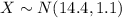
Where
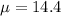`\mu=14.4` and
`\sigma=1.1`

For this part we want to find a value a, such that we satisfy this condition:

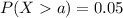`P(X>a)=0.05` (a)

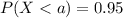`P(X<a)=0.95` (b)

We can find a quantile in the normal standard distribution who accumulates 0.95 of the area on the left and 0.05 of the area on the right it's z=1.64

Using this value we can set up the following equation:

`P(X<a)=P((X-\mu)/(\sigma)<(a-\mu)/(\sigma))=0.95`

`P(z<(a-\mu)/(\sigma))=0.95`

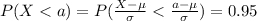
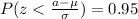
And we have:

`z=1.64<(a-14.4)/(1.1)`

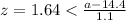
And if we solve for a we got

`a=14.4 +1.64*1.1=16.204`

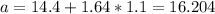
The 95th percentile of the hip breadth of adult men is 16.2 inches.