Question

two sides of a parallelogram meet at an angle of 50 degrees. If the length of one side is 3 meters and the length of the other side is 5 meters, find the length of the longest diagonal and the angles that it forms with each of the given sides.

Answer 1

The longer diagonal has a length of 7.3 meters.

The angles are 31.65° and 18.35°

Explanation:

If one angle of the parallelogram is 50°, another angle is also 50° and the other two angles are the supplement of this angle. so the other three angles are:

50°, 130° and 130°.

The longer diagonal will be the one opposite to the bigger angle (130°), and this diagonal divides the parallelogram in two triangles.

Using the law of cosines in one of these two triangles, we have:

`diagonal^2 = a^2 + b^2 - 2ab*cos(130\°)`

`diagonal^2 = 3^2 + 5^2 - 2*3*5*(-0.6428)`

`diagonal^2 = 53.284`

`diagonal = 7.3\ meters`

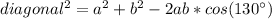
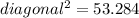
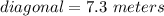
So the longer diagonal has a length of 7.3 meters.

To find the angles that this diagonal forms with the sides, we can use the law of sines:

`a / sin(A) = b/sin(B)`

`5 / sin(A) = diagonal / sin(130)`

`sin(A) = 5 * sin(130) / 7.3`

`sin(A) = 0.5247`

`A = 31.65\°`

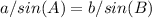
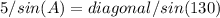
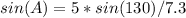
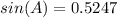
The other angle is B = 50 - 31.65 = 18.35°

Please check the image attached for better comprehension.