Answer:
[CH₂Cl₂] = 7.07x10⁻² M
[CH₄] = 0.319 M
[CCl₄] = 0.164 M
Step-by-step explanation:
The equilibrium reaction is the following:
2CH₂Cl₂(g) ⇄ CH₄(g) + CCl₄(g)
The equilibrium constant of the above reaction is:
![K = ([CH_(4)][CCl_(4)])/([CH_(2)Cl_(2)]^(2)) = (0.173 M*0.173 M)/((5.35 \cdot 10^(-2) M)^(2)) = 10.5](https://img.qammunity.org/2021/formulas/chemistry/college/s0wmjw6mgmba1knsrhts93zuj4m8pobt0w.png)
When 0.155 mol of CH₄(g) is added to the flask we have the following concentration of CH₄:
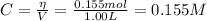
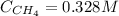
Now, the concentrations at the equilibrium are:
2CH₂Cl₂(g) ⇄ CH₄(g) + CCl₄(g)
5.35x10⁻² - 2x 0.328 + x 0.173 + x
![K = ([CH_(4)][CCl_(4)])/([CH_(2)Cl_(2)]^(2)) = ((0.328 + x)(0.173 + x))/((5.35 \cdot 10^(-2) - 2x)^(2))](https://img.qammunity.org/2021/formulas/chemistry/college/aixncs3rs6iumg9cl2x0ewqx9t7rqduwig.png)
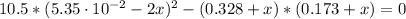
Solving the above equation for x:
x₁ = 0.076 and x₂ = -0.0086
Hence, the concentration of the three gases once equilibrium has been reestablished is:
[CH₂Cl₂] = 5.35x10⁻² - 2(-0.0086) = 7.07x10⁻² M
[CH₄] = 0.328 + (-0.0086) = 0.319 M
[CCl₄] = 0.173 + (-0.0086) = 0.164 M
We took x₂ value because the x₁ value gives a negative CH₂Cl₂ concentration.
I hope it helps you!