Complete Question
From the mid-1960's to the early 1990's, there was a slow but steady decline in SAT scores. For example, take the Verbal SAT. The average in 1967 was about 543; by 1994, the average was down to about 499. However, the SD stayed close to 110. The drop in average has a large effect on the tails of the distribution.
Estimate the percentage of students scoring over 700 on 1967.
A 0.7%
B 7%
C 7.67%
D 7.6%
Answer:
The correct option is D
Explanation:
From the question we are told that
The average SAT score in 1967 is
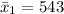
The standard deviation of score in 1967 is
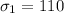
The average SAT score in 1994 is

The standard deviation of score in 1967 is
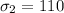
The percentage of students scoring over 700 on 1967 is mathematically represented as
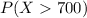
Where X is the random variable representing score of student above 700
Now normalizing the above probability we have
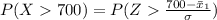
substituting values
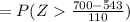
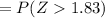
Form the normalized z table
= 0.076
= 7.6 %