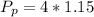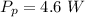Question

You measure the power delivered by a battery to be 1.15 W when it is connected in series with two equal resistors. How much power will the same battery deliver if the resistors are now connected in parallel across it

Answer 1

The power is
`P_p = 4.6 \ W`

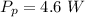
Step-by-step explanation:

From the question we are told that

The power delivered is
`P_(s) = 1.15 \ W`

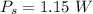
Let it resistance be denoted as R

The resistors are connected in series so the equivalent resistance is

`R_(eqv) = R+ R = 2 R`

Considering when it is connected in series

Generally power is mathematically represented as

`P_s = V * I`

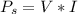
Here I is the current which is mathematically represented as

`I = (V)/(2R)`

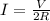
The power becomes

`P_s = V * (V)/(2R)`

`P_s = (V^2)/(2R)`

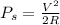
substituting value

`1.15 = (V^2)/(2R)`

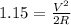
Considering when resistance is connected in parallel

The equivalent resistance becomes

`R_(eqv) = (R)/(2)`

So The current becomes

`I = (V)/((R)/(2) ) = (2V)/(R)`

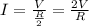
And the power becomes

`P_p = V * (2V)/(R) = (2V^2)/(R) = (4 V^2)/(2 R) = 4 * P_s`

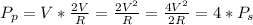
substituting values

`P_p = 4 * 1.15`

`P_p = 4.6 \ W`