Question

What is the surface area of the right cone below?

slanted height of 13 and radius of 4

A. 5277 units

B. 5477 units2

C. 687 units2

D. 104 units2

Answer 1

The surface area of the right cone with slanted height of 13 and radius of 4 is 221.06 square units.

What is the surface area of the right cone below?

Surface area of a right cone

`=\pi \: r(r + \sqrt{ {h}^(2) + {r}^(2) })`

`=3.14 *4 (4 + \sqrt{ {13}^(2) + {4}^(2) })`

`=12.56 (4 + \sqrt{ {169} + {16} })`

`=12.56 (4 + \sqrt{ {185} })`

`=12.56 (4 + 13.60 )`

`= 12.56(17.60)`

`= 221.06`

Therefore, surface area equals 221.06 square units.

Answer 2

213.69 units

Explanation:

We have to first find the height of the cone.

We can use Pythagoras rule because the slant height, height and radius of a cone all form a right angled triangle:

`h^2 = a^2 + b^2`

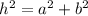
where h = hypotenuse

a and b = the other two sides of the triangle

The radius is 4 units and the slant height is 13 units:

`13^2 = a^2 + 4^2\\\\169 = a^2 + 16\\\\a^2 = 169 - 16 = 153\\\\a = √(153)\\\\a = 12.37units`

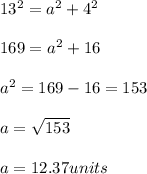
The height of the cone is 12.37 units.

The surface area of a cone is given as:

SA =
`\pi r (r + √(h^2 + r^2) )`

`SA = \pi * 4(4 + √(12.37^2 + 4^2) )\\\\SA = 12.57(4 + √(13^2) )\\SA = 12.57 (4 + 13)\\\\SA = 12.57(17)\\\\SA = 213.69 units`

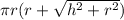
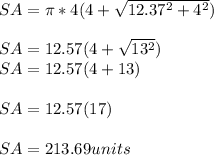
The surface area of the cone is 213.69 units.