Question

A statistics professor receives an average of five e-mail messages per day from students. Assume the number of messages approximates a Poisson distribution. What is the probability that on a randomly selected day she will have five messages

Answer 1

The probability that on a randomly selected day the statistics professor will have five messages is 0.1755.

Explanation:

Let the random variable X represent the number of e-mail messages per day a statistics professor receives from students.

The random variable is approximated by the Poisson Distribution with parameter λ = 5.

The probability mass function of X is as follows:

`P(X=x)=(e^(-5)\cdot 5^(x))/(x!);\ x=0,1,2,3...`

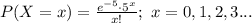
Compute the probability that on a randomly selected day she will have five messages as follows:

`P(X=5)=(e^(-5)\cdot 5^(5))/(5!)`

`=(0.006738* 3125)/(120)\\\\=0.17546875\\\\\approx 0.1755`

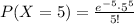
Thus, the probability that on a randomly selected day the statistics professor will have five messages is 0.1755.