Question

The length of time for one individual to be served at a cafeteria is an exponential random variable with mean of 5 minutes. Assume a person has waited for at least 3 minutes to be served. What is the probability that the person will need to wait at least 7 minutes total

Answer 1

44.93% probability that the person will need to wait at least 7 minutes total

Explanation:

To solve this question, we need to understand the exponential distribution and conditional probability.

Exponential distribution:

The exponential probability distribution, with mean m, is described by the following equation:

`f(x) = \mu e^(-\mu x)`

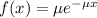
In which
`\mu = (1)/(m)` is the decay parameter.

The probability that x is lower or equal to a is given by:

`P(X \leq x) = \int\limits^a_0 {f(x)} \, dx`

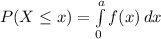
Which has the following solution:

`P(X \leq x) = 1 - e^(-\mu x)`

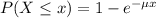
The probability of finding a value higher than x is:

`P(X > x) = 1 - P(X \leq x) = 1 - (1 - e^(-\mu x)) = e^(-\mu x)`

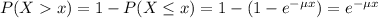
Conditional probability:

We use the conditional probability formula to solve this question. It is

`P(B|A) = (P(A \cap B))/(P(A))`

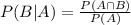
In which

P(B|A) is the probability of event B happening, given that A happened.

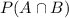`P(A \cap B)` is the probability of both A and B happening.

P(A) is the probability of A happening.

The length of time for one individual to be served at a cafeteria is an exponential random variable with mean of 5 minutes

This means that
`m = 5, \mu = (1)/(5) = 0.2`

Assume a person has waited for at least 3 minutes to be served. What is the probability that the person will need to wait at least 7 minutes total

Event A: Waits at least 3 minutes.

Event B: Waits at least 7 minutes.

Probability of waiting at least 3 minutes:

`P(A) = P(X > 3) = e^(-0.2*3) = 0.5488`

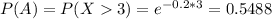
Intersection:

The intersection between waiting at least 3 minutes and at least 7 minutes is waiting at least 7 minutes. So

`P(A \cap B) = P(X > 7) = e^(-0.2*7) = 0.2466`

What is the probability that the person will need to wait at least 7 minutes total

`P(B|A) = (0.2466)/(0.5488) = 0.4493`

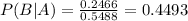
44.93% probability that the person will need to wait at least 7 minutes total